Atoms and the Periodic Table III: The Wave Function
Before we discuss more thoroughly about the H atom as a wave function, it might be better to have a look the older atomic model.
Firstly, the Rutherford's model was not completely incorrect about the structure. Its model was built from its observation when a gold foil was shot by alpha particle. Rutherford's model only stated that electron is orbiting a nucleus, so the interaction is purely electrostatic interaction. However, the weakness of this model is the collapsing orbit of an electron. Since an electron is attracted by force from nucleus, so it is accelerating motion, and as the energy as the result of movement increases, it will collapse to the nucleus. Fortunately, this phenomena does not happen. Moreover, this structure also does not agree with the observation at the spectra. In this model it is expected to have a broad region of spectra, but in reality it appears as the line spectra.
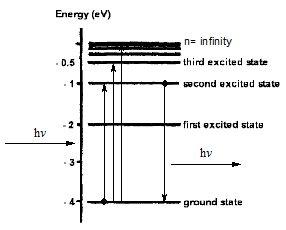
After that, the Bohr's model is slightly promising than Rutherford's. In Bohr's model, it is assumed that electron occupies at certain orbit, more precisely at specific orbit, which implies the atomic radius. Since electron occupies at certain orbit, it also said the energy in every orbit is constant, so every electron occupies a certain level of energy. This model is agree with the observation of spectra which produces a certain pattern of line and also this model implies what we called a quantised orbit. Besides that, this model also can explain the electron transition phenomena and also it can predict the Rydberg's constant precisely. However, this model cannot explain why every electron occupy a certain energy level.
To provide the reason of this phenomenon, a new approach of mechanics theory should be formulated. In classical mechanics which base on 3 Newton's laws can be applied only at a large system. However, for a small object in order nanometre such as electron and proton a new approach of mechanics should be made. Another type of mechanics was formulated and known as quantum mechanics. In quantum mechanics, a matter not only behave as a particle, but also it has wave properties. This was shown by using electron diffraction. If electron behave as particle, two bright line should appear on diffraction, but a dark-bright line appeared at the screen, so the electron also have wave properties
According de Broglie equation above, from the wavelength and momentum it also implies the energy of the particle, and the wavelength implies the concept of quantised orbit. Therefore, if we would like to predict the electron behaviour, we need to be able to find or formulate the wave function. As it is stated before, the classical mechanics is based on 3 Newton's Laws, but the quantum mechanics is based on 1 base law which is Schrodinger's equation. However, before we discuss about Schrodinger equation, it might be better to see the problems as electron has wave properties.
The problems of electron has wave properties implies the uncertainty about the electron itself. As we know, the wave propagate from -∞ up to ∞ for a certain wavelength and the result of this we cannot precisely and accurately where the exact position of electron is. As the wave propagates, the electron can be along at the wave. Therefore, in a wave at certain wavelength you cannot tell where the electron is. Second problem, if we can tell where the exact position of electron is, you will be lack of information (or no information at all) about the wavelength. Therefore, if the wavelength is unknown, the momentum of the electron cannot be found.
From those arguments, we can formulate that relates both uncertainty, which is known as Heisenberg's uncertainty principle:
From the uncertainty principle, we cannot find the exact position or the momentum simultaneously and accurately. What we can do from those uncertainties is we can find the probability of electron to be at given position. Therefore, Schrodinger equation take role to solve this problem.
Basically, Schrodinger equation has 2 main variable, the energy of the electron which depends on the energy level (n) and the wave function for given n. The wave function itself is a function of position. Therefore, we can have a pair of solution of energy and wave function and the Schrodinger equation is:
Where n is a quantum number or set of quantum numbers, H is the hamiltonian function, E is energy, and ψ is the wave function.
This equation is a time-independent equation and also it can have several wave function that have same energy (degeneracy). Therefore, the wave function is a one-dimensional function which the value run from ∞, 0, and -∞. Moreover, if we take the absolute value of wave function, square it, and multiply with a small change of distance (dx), so we can find the probability of electron at the certain place from x to x + dx.
Moreover, the absolute value square of wave function is the electron distribution function.
Firstly, the Rutherford's model was not completely incorrect about the structure. Its model was built from its observation when a gold foil was shot by alpha particle. Rutherford's model only stated that electron is orbiting a nucleus, so the interaction is purely electrostatic interaction. However, the weakness of this model is the collapsing orbit of an electron. Since an electron is attracted by force from nucleus, so it is accelerating motion, and as the energy as the result of movement increases, it will collapse to the nucleus. Fortunately, this phenomena does not happen. Moreover, this structure also does not agree with the observation at the spectra. In this model it is expected to have a broad region of spectra, but in reality it appears as the line spectra.
After that, the Bohr's model is slightly promising than Rutherford's. In Bohr's model, it is assumed that electron occupies at certain orbit, more precisely at specific orbit, which implies the atomic radius. Since electron occupies at certain orbit, it also said the energy in every orbit is constant, so every electron occupies a certain level of energy. This model is agree with the observation of spectra which produces a certain pattern of line and also this model implies what we called a quantised orbit. Besides that, this model also can explain the electron transition phenomena and also it can predict the Rydberg's constant precisely. However, this model cannot explain why every electron occupy a certain energy level.
Then, de Broglie stated that a particle which has momentum (particle properties) should also have wave properties which the wave should fit in a system. It can be formulated as:
 |
| No information for exact position |
 |
| No exact information for momentum |
Basically, Schrodinger equation has 2 main variable, the energy of the electron which depends on the energy level (n) and the wave function for given n. The wave function itself is a function of position. Therefore, we can have a pair of solution of energy and wave function and the Schrodinger equation is:
This equation is a time-independent equation and also it can have several wave function that have same energy (degeneracy). Therefore, the wave function is a one-dimensional function which the value run from ∞, 0, and -∞. Moreover, if we take the absolute value of wave function, square it, and multiply with a small change of distance (dx), so we can find the probability of electron at the certain place from x to x + dx.
Comments